欧拉公式的三种证明方法:导数、幂级数、极坐标
目录
- 欧拉公式(Euler's formula)
- 证明
- 方法1: 使用导数
- 方法2: 使用幂级数
- 方法3: 使用 极坐标
- 虚数转极坐标背景知识
- 开始证明
欧拉公式(Euler’s formula)
e i x = cos x + i sin x e^{ix} = \cos x + i\sin x eix=cosx+isinx
证明
方法1: 使用导数
如果函数 f ( x ) f(x) f(x)的导数满足对任意的 x x x, f ′ ( x ) = 0 f'(x)=0 f′(x)=0,且存在 x ′ x' x′使 f ( x ′ ) = C f(x')=C f(x′)=C,则该函数为常数函数 y = C y=C y=C,其中 C C C为常数。
下面根据这个结论证明欧拉公式:
令 f ( θ ) = cos θ + i sin θ e i θ = e − i θ ( cos θ + i sin θ ) f(\theta) = \frac{\cos\theta + i\sin\theta}{e^{i\theta}} = e^{-i\theta} \left(\cos\theta + i \sin\theta\right) f(θ)=eiθcosθ+isinθ=e−iθ(cosθ+isinθ)
∵ \because ∵ f ′ ( θ ) = e − i θ ( i cos θ − sin θ ) − i e − i θ ( cos θ + i sin θ ) = 0 f'(\theta) = e^{-i\theta} \left(i\cos\theta - \sin\theta\right) - ie^{-i\theta} \left(\cos\theta + i\sin\theta\right) = 0 f′(θ)=e−iθ(icosθ−sinθ)−ie−iθ(cosθ+isinθ)=0
∴ \therefore ∴ f ( θ ) f(\theta) f(θ)是常数
∵ \because ∵ f ( 0 ) = 1 f(0)=1 f(0)=1
∴ \therefore ∴ 对任意 θ \theta θ,都有 f ( θ ) = 1 f(\theta)=1 f(θ)=1是常数
∴
\therefore
∴
e
i
x
=
cos
x
+
i
sin
x
e^{ix} = \cos x + i\sin x
eix=cosx+isinx
■
方法2: 使用幂级数
本方法使用麦克劳林级数(Maclaurin Series)进行证明。
-
关于虚数 i i i的一些事实:
i 0 = 1 , i 1 = i , i 2 = − 1 , i 3 = − i , i 4 = 1 , i 5 = i , i 6 = − 1 , i 7 = − i ⋮ ⋮ ⋮ ⋮ \begin{align} i^0 &= 1, & i^1 &= i, & i^2 &= -1, & i^3 &= -i, \\ i^4 &= 1, & i^5 &= i, & i^6 &= -1, & i^7 &= -i \\ &\vdots & &\vdots & &\vdots & &\vdots \end{align} i0i4=1,=1,⋮i1i5=i,=i,⋮i2i6=−1,=−1,⋮i3i7=−i,=−i⋮ -
s i n ( x ) sin(x) sin(x)和 c o s ( x ) cos(x) cos(x)的麦克劳林展开:
s i n ( x ) = 1 − x 2 2 ! + x 4 4 ! − x 6 6 ! + x 8 8 ! − ⋯ sin(x) = 1 - \frac{x^2}{2!} + \frac{x^4}{4!} - \frac{x^6}{6!} + \frac{x^8}{8!} - \cdots sin(x)=1−2!x2+4!x4−6!x6+8!x8−⋯
c o s ( x ) = i ( x − x 3 3 ! + x 5 5 ! − x 7 7 ! + ⋯ ) cos(x) = i\left( x - \frac{x^3}{3!} + \frac{x^5}{5!} - \frac{x^7}{7!} + \cdots \right) cos(x)=i(x−3!x3+5!x5−7!x7+⋯) -
e x e^x ex的麦克劳林展开:
e i x = 1 + i x + ( i x ) 2 2 ! + ( i x ) 3 3 ! + ( i x ) 4 4 ! + ( i x ) 5 5 ! + ( i x ) 6 6 ! + ( i x ) 7 7 ! + ( i x ) 8 8 ! + ⋯ = 1 + i x − x 2 2 ! − i x 3 3 ! + x 4 4 ! + i x 5 5 ! − x 6 6 ! − i x 7 7 ! + x 8 8 ! + ⋯ = ( 1 − x 2 2 ! + x 4 4 ! − x 6 6 ! + x 8 8 ! − ⋯ ) + i ( x − x 3 3 ! + x 5 5 ! − x 7 7 ! + ⋯ ) = cos x + i sin x \begin{align} e^{ix} &= 1 + ix + \frac{(ix)^2}{2!} + \frac{(ix)^3}{3!} + \frac{(ix)^4}{4!} + \frac{(ix)^5}{5!} + \frac{(ix)^6}{6!} + \frac{(ix)^7}{7!} + \frac{(ix)^8}{8!} + \cdots \\[8pt] &= 1 + ix - \frac{x^2}{2!} - \frac{ix^3}{3!} + \frac{x^4}{4!} + \frac{ix^5}{5!} - \frac{x^6}{6!} - \frac{ix^7}{7!} + \frac{x^8}{8!} + \cdots \\[8pt] &= \left( 1 - \frac{x^2}{2!} + \frac{x^4}{4!} - \frac{x^6}{6!} + \frac{x^8}{8!} - \cdots \right) + i\left( x - \frac{x^3}{3!} + \frac{x^5}{5!} - \frac{x^7}{7!} + \cdots \right) \\[8pt] &= \cos x + i\sin x \end{align} eix=1+ix+2!(ix)2+3!(ix)3+4!(ix)4+5!(ix)5+6!(ix)6+7!(ix)7+8!(ix)8+⋯=1+ix−2!x2−3!ix3+4!x4+5!ix5−6!x6−7!ix7+8!x8+⋯=(1−2!x2+4!x4−6!x6+8!x8−⋯)+i(x−3!x3+5!x5−7!x7+⋯)=cosx+isinx
所以欧拉公式得证。■
方法3: 使用 极坐标
虚数转极坐标背景知识
参考:所有复数都可以用极坐标表示
设
r
r
r和
θ
θ
θ是对应于非零复数
z
=
x
+
i
y
z=x+iy
z=x+iy的点(x,y)的极坐标, 因为
x
=
r
c
o
s
θ
x=rcosθ
x=rcosθ,
y
=
r
s
i
n
θ
y=rsinθ
y=rsinθ ,
z
z
z可以被写成极坐标的形式:
z
=
r
(
c
o
s
θ
+
i
s
i
n
θ
)
z=r(cos\theta+isin\theta)
z=r(cosθ+isinθ)。
比如
3
+
4
i
3+4i
3+4i的极坐标表示如下(虚数转极坐标网站链接):
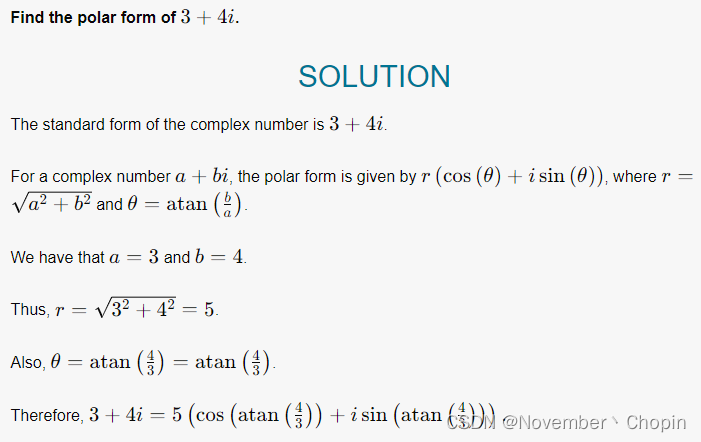
开始证明
设
e
i
x
=
r
(
cos
θ
+
i
sin
θ
)
\begin{align} e^{ix} = r \left(\cos \theta + i \sin \theta\right) \end{align}
eix=r(cosθ+isinθ)如果证明(8)式的
r
=
1
r=1
r=1,则欧拉公式得证。
对(8)式两边同时求导,可得:
i
e
i
x
=
(
cos
θ
+
i
sin
θ
)
d
r
d
x
+
r
(
−
sin
θ
+
i
cos
θ
)
d
θ
d
x
\begin{align} i e ^{ix} = \left(\cos \theta + i \sin \theta\right) \frac{dr}{dx} + r \left(-\sin \theta + i \cos \theta\right) \frac{d\theta}{dx} \end{align}
ieix=(cosθ+isinθ)dxdr+r(−sinθ+icosθ)dxdθ
用
r
(
cos
θ
+
i
sin
θ
)
r \left(\cos \theta + i \sin \theta\right)
r(cosθ+isinθ)代替
e
i
x
e^{ix}
eix并化简可得:
(
c
o
s
θ
d
r
d
x
−
r
s
i
n
θ
d
θ
d
x
+
r
s
i
n
θ
)
+
i
(
s
i
n
θ
d
r
d
x
−
r
c
o
s
θ
d
θ
d
x
+
r
c
o
s
θ
)
=
0
\begin{align} (cos\theta {\frac {dr} {dx}}-rsin\theta {\frac {d\theta} {dx}}+rsin\theta)+ i(sin\theta {\frac {dr} {dx}}-rcos\theta {\frac {d\theta} {dx}}+rcos\theta)=0 \end{align}
(cosθdxdr−rsinθdxdθ+rsinθ)+i(sinθdxdr−rcosθdxdθ+rcosθ)=0
因此(10)式的实部和虚部均等于0:
c
o
s
θ
d
r
d
x
−
r
s
i
n
θ
d
θ
d
x
+
r
s
i
n
θ
=
0
s
i
n
θ
d
r
d
x
−
r
c
o
s
θ
d
θ
d
x
+
r
c
o
s
θ
=
0
\begin{align} cos\theta {\frac {dr} {dx}}-rsin\theta {\frac {d\theta} {dx}}+rsin\theta=0 \\ sin\theta {\frac {dr} {dx}}-rcos\theta {\frac {d\theta} {dx}}+rcos\theta=0 \end{align}
cosθdxdr−rsinθdxdθ+rsinθ=0sinθdxdr−rcosθdxdθ+rcosθ=0
(11),(12)两式可解得 d r d x = 0 {\frac {dr} {dx}}=0 dxdr=0, d θ d x = 1 {\frac {d\theta} {dx}}=1 dxdθ=1,因此 r r r是一个常数C1, θ \theta θ是 x + C 2 x+C2 x+C2。
因为
e
0
i
=
1
e^{0i}=1
e0i=1,可得
r
=
C
1
=
1
r=C1=1
r=C1=1,进而通过
1
=
c
o
s
θ
+
i
s
i
n
θ
1=cos\theta+isin\theta
1=cosθ+isinθ得出
θ
=
0
+
C
2
=
0
\theta=0+C2=0
θ=0+C2=0,所以C1=1,C2=0,即
r
=
1
r=1
r=1,
θ
=
x
\theta=x
θ=x,因此:
e
i
x
=
1
(
cos
x
+
i
sin
x
)
=
cos
x
+
i
sin
x
.
e^{ix} = 1(\cos x +i \sin x) = \cos x + i \sin x.
eix=1(cosx+isinx)=cosx+isinx.
欧拉公式得证。■
